An equilateral triangle and a parallelogram lie on the same base and between the same parallel lines. The area of the parallelogram is 200*root(3) sq cm. What is the length of the side of the triangle?Explanation: Two triangles with the same base and between the same parallel lines will have equal areas. Likewise, a triangle and a parallelogram with the same base and between … [Read more...] about GRE Geometry – same base, same height
GRE Geometry, Solid Geometry, Coordinate Geometry
GRE Geometry – Planet Circles
Consider a planet P1 (like earth) that is spherical in shape. It has an atmospheric layer around it that also takes the same shape as that of the planet. An adventurist launches a rocket from a neighboring planet P2 to this planet P1. The rocket cuts the atmospheric layer at point A, grazes the planet P1 and goes out of the atmospheric layer at point B. If AB = 100 km, what is … [Read more...] about GRE Geometry – Planet Circles
GRE Geometry – Chords and Circles
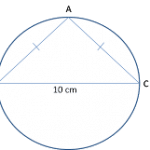
A, B and C are three points on a circle. AB, BC and AC are three chords where AB = AC. If the maximum length of BC is 10 cm, what is the area of triangle ABC? Explanation: If BC, which is a chord, should have the maximum length then it should be the diameter. And since angle(BAC) is the angle subtended by the diameter at the circumference, it should be 90 degrees. And we … [Read more...] about GRE Geometry – Chords and Circles
Solid Geometry – Sphere and Cylinder Volumes
A cylindrical vessel is filled with water up to some height. When a sphere of diameter 8 cm is dropped into the cylinder the water level rises by half of the initial level. When a sphere of diameter 16 cm is dropped the water level rises by some other value. What percentage of this new height is the initial level of water? Answer: 20% Explanation: Let the radius of the … [Read more...] about Solid Geometry – Sphere and Cylinder Volumes
Solid Geometry Mensuration – Circles
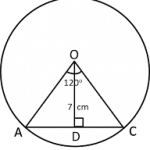
Chord AC at a distance of 7 cm from the center of a circle subtends an angle of 120 degrees at the center. Find the area of major segment. Answer: 1232/3 + 49*root(3) Explanation: In triangle OCD, Angle (COD) = 60 degrees Angle (OCD) = 30 degrees Hence tan(OCD) = OD/CD CD = 7*root(3) Sin(OCD) = OD/OC OC = 14 Hence AC = 14*root(3) Area of triangle AOC = … [Read more...] about Solid Geometry Mensuration – Circles