8 directors, the vice chairman and the chairman are to be seated around a circular table. If the chairman should sit between a director and the vice chairman, in how many ways can they be seated? Answer: 8! * 2 Explanation: Let us consider the vice chairman and the chairman together as one entity. Hence, 8 directors + this entity can be arranged circularly in (9-1)! ways. … [Read more...] about Permutation Rearrangement
Number Properties – Factorial & Remainders
If p = 1! + (2x2!) + (3x3!) + (4x4!)...... + (10x10!), what is the remainder when p+2 is divided by 11!? Answer: 1 Explanation: p = 1! + (2x2!) + (3x3!) + (4x4!)...... + (10x10!) p = (1x1!) + (2x2!) + (3x3!) + (4x4!)...... + (10x10!) But, (1x1!) = 2! – 1! (2x2!) = 3! – 2! (3x3!) = 4! – 3! And so on. Hence, p = 2! – 1! + 3! – 2! + 4! – 3! + 5! – 4! + 6! – 5! + 7! – 6! … [Read more...] about Number Properties – Factorial & Remainders
Statistics – Mean, Median, Range
The maximum mark in an examination is 100 and the minimum is 0. The average mark of seven students such that no two of them have scored the same marks is 88. If the median score is 92 and all the marks are integers, what is the maximum possible difference between the largest and the smallest mark obtained by these seven students? Answer: 54 Explanation: Average mark of … [Read more...] about Statistics – Mean, Median, Range
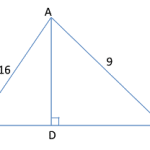
Geometry Triangle – Equating Areas
In a triangle ABC, AB = 16, AC = 9. Find the length of the altitude to BC if the circumradius is 9. Answer: 8 Explanation: Consider the figure below. We know that, Area of a triangle = abc/4R where a,b,c are the sides of the triangle and R is the circumradius. Hence, (AB x BC x AC)/4R = (1/2) x BC x AD (16 x BC x 9)/36 = (1/2) x BC x AD Therefore, AD = 8. … [Read more...] about Geometry Triangle – Equating Areas
Algbera Practice: Functions
A function is defined as f(x+2) = 3 + f(x) when x is even f(x+2) = x + f(x) when x is odd f(1) = 4 and f(2) = 3 Find f(f(f(1))) + f(f(f(2))) Answer: 17 Explanation: f(f(f(1))) + f(f(f(2))) = f(f(4)) + f(f(3)) Now f(4) can be found by using the definition of the function when x is even. Take x = 2. f(2+2) = 3 + f(2) f(4) = 3 + 3 f(4) = 6 Likewise, f(3) can be … [Read more...] about Algbera Practice: Functions