Solve: (a) 7^(4-16x) > 1 (b) 2/(x-1) < 1 (a) For any a^b > 1, if a > 1 then b should be greater than 0. Hence 4 – 16x > 0 Therefore, x<1/4. (b) 2/(x-1) < 1 2/(x-1) - 1< 0 [2-(x-1)]/(x-1) < 0 (3-x)/(x-1) < 0 (x-3)/(x-1) > 0 Hence ‘x’ lies outside the roots ‘1’ and ‘3’. Therefore, x € (-∞ , 1) U (3, ∞) … [Read more...] about Algebra – Inequalities and Exponents
Solid Geometry – Sphere and Cylinder Volumes
A cylindrical vessel is filled with water up to some height. When a sphere of diameter 8 cm is dropped into the cylinder the water level rises by half of the initial level. When a sphere of diameter 16 cm is dropped the water level rises by some other value. What percentage of this new height is the initial level of water? Answer: 20% Explanation: Let the radius of the … [Read more...] about Solid Geometry – Sphere and Cylinder Volumes
Solid Geometry Mensuration – Circles
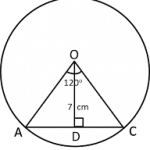
Chord AC at a distance of 7 cm from the center of a circle subtends an angle of 120 degrees at the center. Find the area of major segment. Answer: 1232/3 + 49*root(3) Explanation: In triangle OCD, Angle (COD) = 60 degrees Angle (OCD) = 30 degrees Hence tan(OCD) = OD/CD CD = 7*root(3) Sin(OCD) = OD/OC OC = 14 Hence AC = 14*root(3) Area of triangle AOC = … [Read more...] about Solid Geometry Mensuration – Circles
Number Properties LCM HCF
If the HCF of two natural numbers whose sum is 216 is 12, how many such pairs exist? Answer: 3 pairs Explanation: Let the two number be 12a and 12b where a and b are relatively prime. Then 12a + 12b = 216 a + b = 18 Now, this boils down to finding out all possible ways of writing 18 as a sum of two natural numbers co-prime to each … [Read more...] about Number Properties LCM HCF
Word Problem – Rates Work and Time
If A and B together can complete a work in 4 days, B and C together in 6 days, C and A together in 5 days, then working individually, who will finish the work in the least time and in how many days? Answer: A, 120/17 days Explanation: Let the rates at which A, B and C work be a, b and c respectively. Therefore, a + b = ¼ b + c = 1/6 c + a = 1/5 Solving for a, b … [Read more...] about Word Problem – Rates Work and Time